Electromagnetic boundary conditions

Boundary-value problems form a broad class of problems in electromagnetism that involve the interface between two different materials. Our fundamental question is: given a field in one material, what is the resulting field in the other?

In both the electric and magnetic cases, we use Gauss’ law and Ampere’s law as a guiding principle to set up our boundary conditions. We can decompose the vector field into normal and tangential components:

$$F_{i,n}=(\hat{n}\cdot {\vec{F}}_i)\hat{n}$$ $$F_{i,t}=\hat{n}\times {\vec{F}}_i$$

This is similar to problems in optics with waves in different media.

Electric field

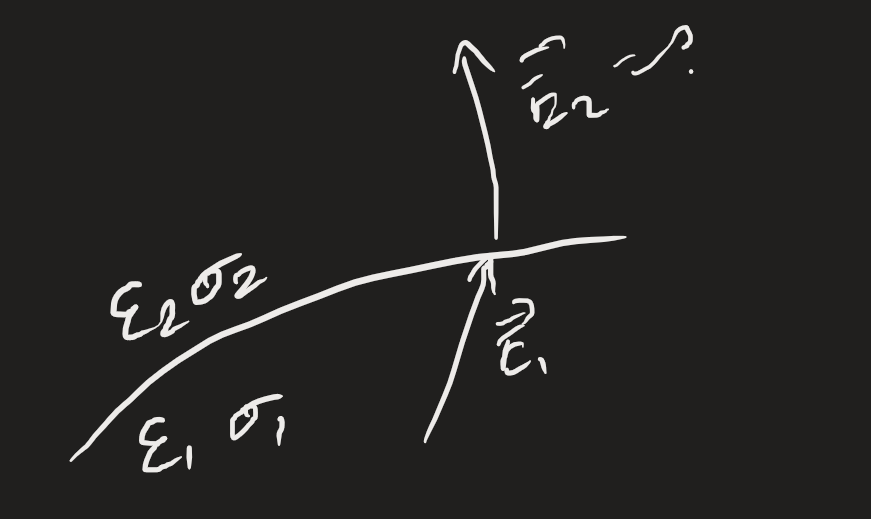The normal components are conditionally continuous:

$$\hat{n}\cdot ({\vec{D}}_2-{\vec{D}}_1)={\rho }_s$$

The tangential component is unconditionally continuous due to Ampere’s law:

$$E_{2,t}=E_{1,t}$$

Special cases

We have two big special cases that simplify our BVP. One is a dielectric to dielectric interface, and the other is with one material as a perfect conductor.

In the dielectric-dielectric interface:

$$D_{2,n}=D_{1,n}$$ $${\vec{E}}_{2,n}=\frac{{ϵ}_1}{{ϵ}_2}{\vec{E}}_{1,n}$$

In the perfect conductor case, ${\vec{E}}_1=0$ (or the other vector), then the boundary condition reduces to:

$$\hat{n}\cdot {\vec{D}}_2={\vec{D}}_{2,n}={\rho }_s$$

Magnetic field

In the magnetic field case, the normal components of the magnetic field are unconditionally continuous:

$$\hat{n}\cdot ({\vec{B}}_2-{\vec{B}}_1)=0$$ $$B_{2,n}=B_{1,n}$$

For the tangential component, we have:

$$\hat{n}\times ({\vec{H}}_2-{\vec{H}}_1)={\vec{J}}_s$$

We have one special case this time. Since surface currents can only exist on the surfaces of perfect conductors and superconductors, at the interface between media with finite conductivities, ${\vec{J}}_s=0$ and:

$$H_{1,t}=H_{2,t}$$

Problem-solving

Okay. We can now tackle some strategies for boundary conditions. First: see if we fall into one of our special cases. If it does, the problem usually falls apart right away. For example, when using Poisson’s equation, if one plate is grounded, we know one field vector is 0.

If we don’t have a special case, we have to do some thinking.

• Figure out which media is medium 1 and 2. This is self-explanatory but it saves some confusion.
• Figure out what the unit vector $\hat{n}$ is.
• Determine where we might have unconditional continuity. Write those components down.
• Evaluate the cross-product or dot product accordingly to find the unknown values.