Trace

We define the trace as the 2D cross-sectional view of a 3D graph, usually considering the intersection space of a plane with a function. We commonly use the $xy$, $yz$, and $xz$ planes.

What does this mean? For an $xy$ plane and a function in ${\mathbb{R}}^3$, we set $z=0$. This usually simplifies it enough for us to understand what we’re graphing.

Let’s use the example $f(x,y)=z=\frac{x^2}{a^2}+\frac{y^2}{b^2}-c$. The $xy$ trace will give us an ellipse. The $xz$ and $yz$ traces will give us an upwards parabola with minimum point at 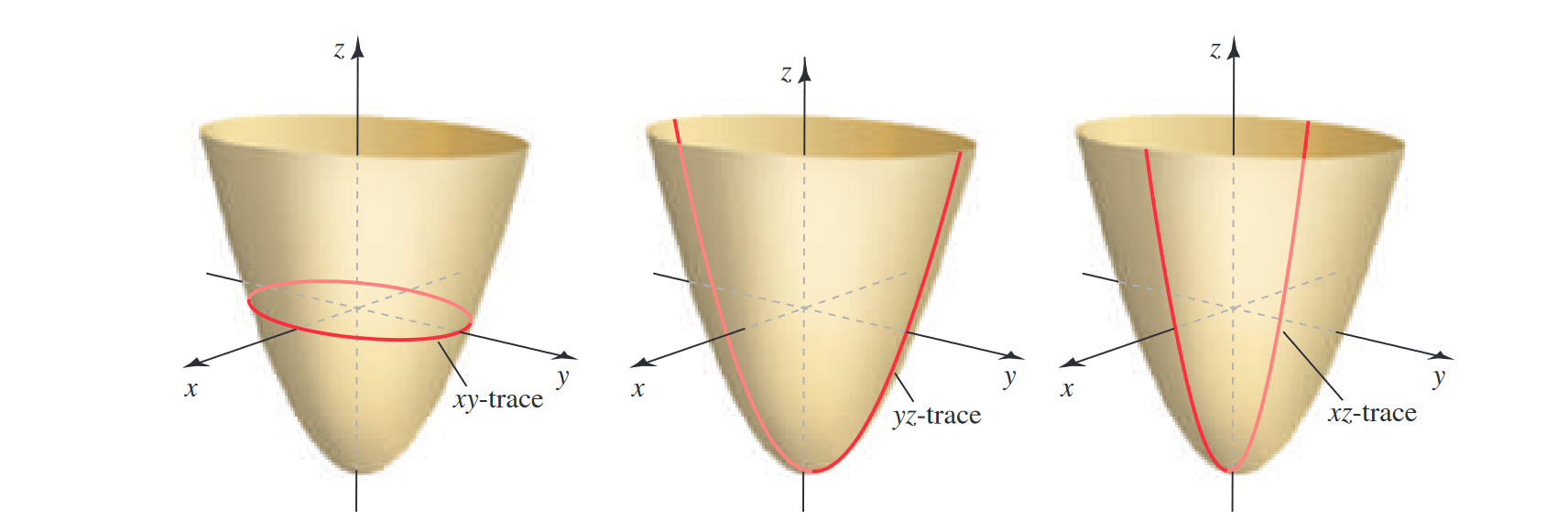$y=-c$. After sketching our traces, we can create a composite sketch of all at once. For example, we would have the three traces above overlaid the $xyz$ axes. Then, we can roughly fill in between. Take note of the domain and range of the functions.