Stress and strain

We test materials based on their physical properties, the most important of which are stress and strain. We’re interested in material properties, but Hooke’s law, $F=k\Delta x$ is sample-size dependent. If two samples are exactly the same material but are different sizes, the larger material appears to be stronger with a higher spring constant. We aim to normalise the behaviour and make measurement sample-size independent.

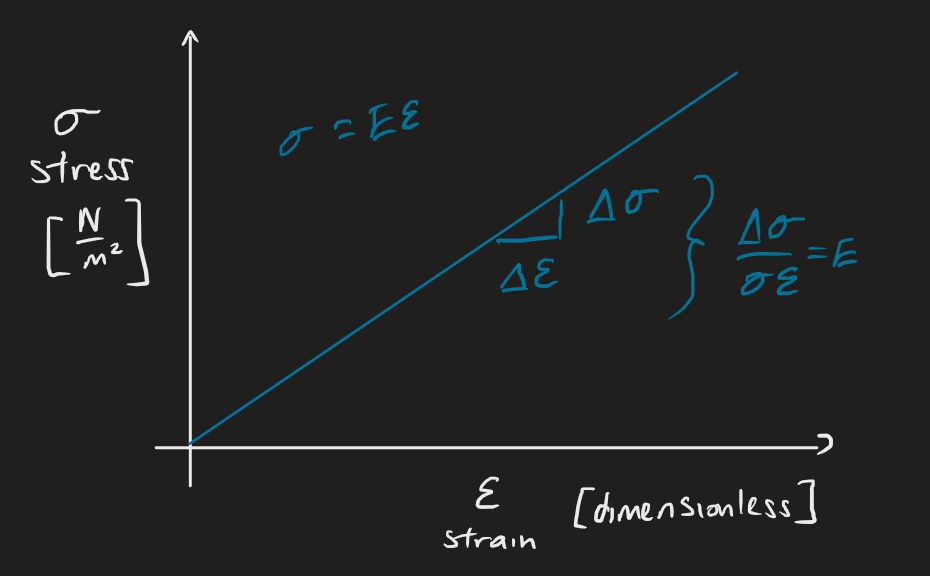
We measure stress ($\sigma$) in pascals (Pa), defined as the applied force divided by the initial cross-sectional area. Strain ($ϵ$) is dimensionless. $\sigma =\frac{F}{A_0}$ $ϵ=\frac{\Delta l}{l_0}$ The slope of a plotted stress versus strain curve is the Young’s modulus, $E$. $E=\frac{\Delta \sigma }{\Delta ϵ}$ Since we test ceramics differently than metals, we use a different formula for a three-point bend: $\sigma =\frac{3Fl}{2w{h}^2}$

Graphically

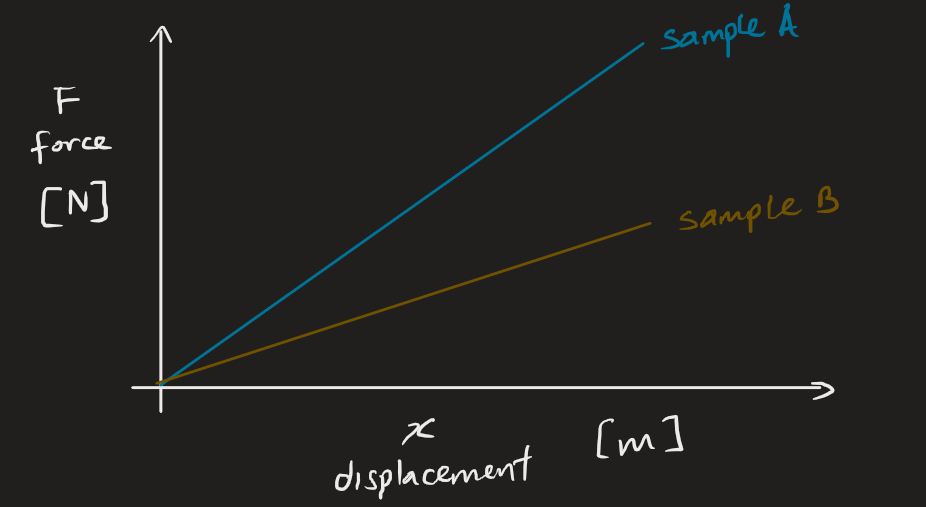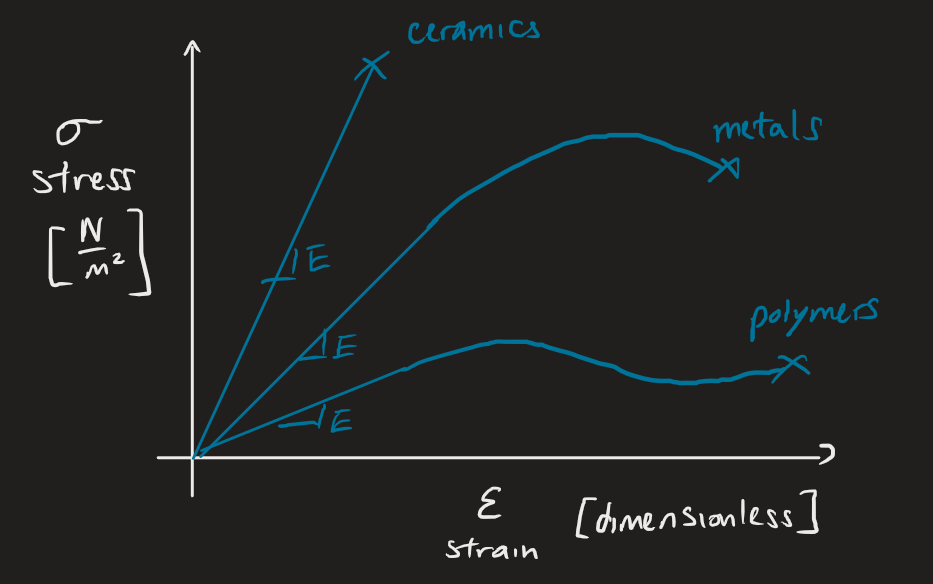
The force-displacement expression of Hooke’s law isn’t very useful for us if we want to figure out material properties. For example, if we have two samples with differing dimensions, we have a different spring constant $k$: Using stress and strain instead. Different materials behave differently.